Difference between revisions of "Gravitational Constant Measurement"
| (2 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| − | == Measurement of | + | == Measurement of Gravitational Constant G == |
| Line 9: | Line 9: | ||
Links: | Links: | ||
[http://www.pasco.com/prodCatalog/AP/AP-8215_gravitational-torsion-balance/ Pasco's Gravitational Torsion Balance] | [http://www.pasco.com/prodCatalog/AP/AP-8215_gravitational-torsion-balance/ Pasco's Gravitational Torsion Balance] | ||
| + | |||
| + | [[File:P-1.jpg]] [[File:P-2.jpg]] | ||
| + | |||
| + | The Gravitational Torsion Balance apparatus is a very accurate device to measure the gravitational constant, G. Isaac Newton deduced the law of universal gravitation without having and evidence of attractive nature between everyday objects. In 1798, Henry Cavendish performed the Torsion Balance experiment with small masses to measure the gravitational constant G. They could then use it to find the mass of the earth. His measurements were most accurate around for about 100 years. | ||
| + | |||
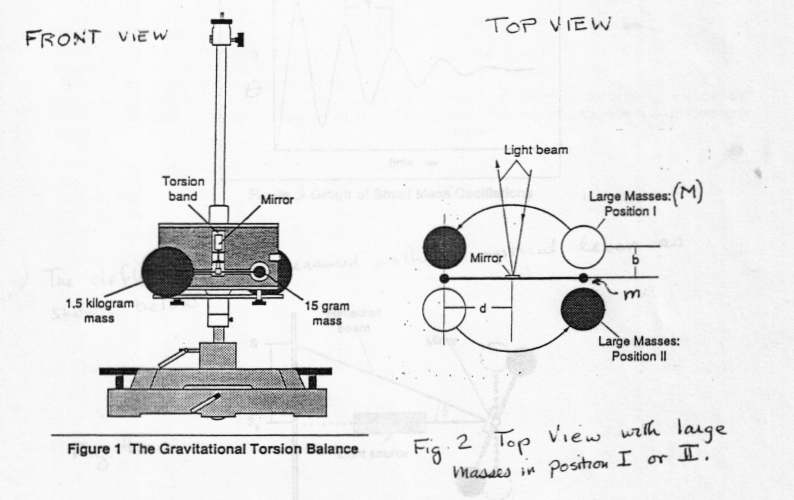
| + | [[File:Picture6.png]] | ||
| + | |||
| + | The pendulum is first aligned vertically using the leveling feet and a leveling sight. I set up the apparatus so that the pendulum mirror is facing a wall about 10 feet away and is normal to the plane of the wall. Using a laser reflecting off the mirror I can use geometry to find the angle displacement and therefore measure things like torque and the period of oscillation by following the laser reflected on the opposite wall. Aligning the pendulum so that at equilibrium, the mirror reflects in the same direction as the reflection of the glass plate in front of the mirror. This process takes many hours due to the long period of oscillation and the slow dampening of the torsion ribbon. After alignment I began the first of three methods. | ||
| + | |||
| + | '''Method I: Measurement by Final Deflection:''' | ||
| + | |||
| + | Starting with the equation of gravitational attraction between to masses one can derive the formula <math>G = (\pi^2)(\Delta S)(b^2)((d^2+(2/5)r^2)/((T^2)(m1)Ld))</math> where <math>\Delta S</math> is the distance between equilibrium points with masses at position I and II shown above. <math>b</math> is the distance between the centers of mass of the big and small masses in position I or II. <math>d</math> is the distance from the center of the small mass to the torsion axis in the pendulum chamber (used to find torque). <math>r</math> is the radius of the small mass. <math>T</math> is the period of oscillation. <math>m1</math> is the mass of the small mass. <math>L</math> id the distance between the mirror and the wall. <math>\Delta S</math> and <math>T</math> can be calculated in the experiment while the rest of the values can be measured. | ||
| + | |||
| + | I this method, I put the masses in position I and wait until the pendulum reaches equilibrium. I then measure the position <math>S1</math> and switch the masses to position II. I then measured the period many times and waited until the pendulum reached equilibrium again and measured <math>S2</math>. With these measurements I calculated <math>G = (5.4 +/-0.4)*10^-11 Nm^2/kg^2</math>. This is within 20% of the accepted value of <math>G = 6.67*10^-11 Nm^2/kg^2</math>. This is a good measurement. | ||
| + | |||
| + | '''Method II: Measurement by Equilibrium Position:''' | ||
| + | |||
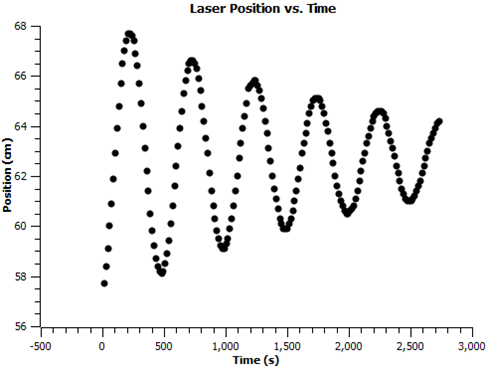
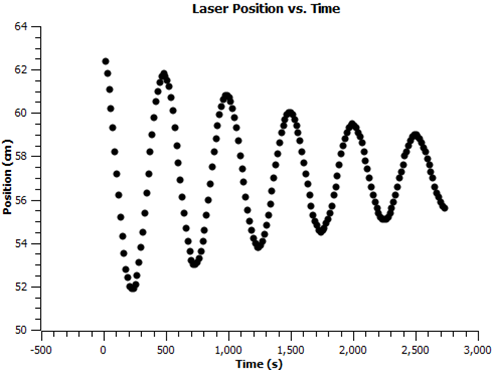
| + | In this method I use the same equation as above. Similarly I measure <math>\Delta S</math> and <math>T</math> and calculate G. First I let the pendulum reach equilibrium with the masses in position I again. As soon as I move the masses to position II I measure the position of the laser on the wall (on a meter stick) every 15 seconds for 45 minutes to an hour. I repeat the process starting with the masses in position II and moving to position I. After plotting the data I could analyze the plots to find <math>S1</math> and <math>S2</math> and thus <math>\Delta S</math> as well as <math>T</math>. | ||
| + | |||
| + | |||
| + | [[File:Picture3.png]] [[File:Picture4.png]] | ||
| + | |||
| + | |||
| + | Plugging in these values I calculate <math>G = (5.5 +/-0.4)*10^-11 Nm^2/kg^2</math> which is within 20% of the accepted value and within 2% of the value measured in method I. This shows that the greatest error is systematic or in the calibration while my different measurement techniques had smaller error. | ||
| + | |||
| + | '''Method III: Measurement by Acceleration:''' | ||
| + | |||
| + | In this method we measure the initial acceleration of the small masses on the pendulum. We have <math>2F = a0 = (2G (m1)(m2))/(b^2)</math> and thus get <math>G = (b^2)(a0)/(2(m1))</math> where <math>a0 = (\Delta S)(d)/((t^2)(L))</math>. After the pendulum reaches equilibrium in position I, I move the masses to position II and measure the position of the laser every 15 seconds for about 2 minutes. By plotting <math>\Delta S</math> over <math>t^2</math> I used a linear fit and found the slope. The plot is below. | ||
| + | |||
| + | |||
| + | [[File:Picture5.png]] | ||
| + | |||
| + | |||
| + | Using this value I solve for <math>G = (4.8 +/-1.2)*10^-9 Nm^2/kg^2</math> which is not as accurate as my previous measurements. I believe the error here is from the speed of me moving the positions of the masses and measuring the initial acceleration. Any delay can lead to measuring a later (and slightly greater) acceleration which gives us a value of G which is too large. | ||
| + | |||
| + | Overall my measurements gave very close values of the gravitational constant G. If i had to change anything, I would calibrate the equilibrium of the pendulum more accurately. I could only get the laser reflected from the mirror on the wall within 5 cm of the laser reflected from the glass plate. Increasing this accuracy should produce better values of G. This process would take many more hours of guess and check work rotating the zero adjust knob and finding the equilibrium until perfect. | ||
Latest revision as of 08:45, 30 March 2015
Measurement of Gravitational Constant G
Links: Pasco's Gravitational Torsion Balance
The Gravitational Torsion Balance apparatus is a very accurate device to measure the gravitational constant, G. Isaac Newton deduced the law of universal gravitation without having and evidence of attractive nature between everyday objects. In 1798, Henry Cavendish performed the Torsion Balance experiment with small masses to measure the gravitational constant G. They could then use it to find the mass of the earth. His measurements were most accurate around for about 100 years.
The pendulum is first aligned vertically using the leveling feet and a leveling sight. I set up the apparatus so that the pendulum mirror is facing a wall about 10 feet away and is normal to the plane of the wall. Using a laser reflecting off the mirror I can use geometry to find the angle displacement and therefore measure things like torque and the period of oscillation by following the laser reflected on the opposite wall. Aligning the pendulum so that at equilibrium, the mirror reflects in the same direction as the reflection of the glass plate in front of the mirror. This process takes many hours due to the long period of oscillation and the slow dampening of the torsion ribbon. After alignment I began the first of three methods.
Method I: Measurement by Final Deflection:
Starting with the equation of gravitational attraction between to masses one can derive the formula where is the distance between equilibrium points with masses at position I and II shown above. is the distance between the centers of mass of the big and small masses in position I or II. is the distance from the center of the small mass to the torsion axis in the pendulum chamber (used to find torque). is the radius of the small mass. is the period of oscillation. is the mass of the small mass. id the distance between the mirror and the wall. and can be calculated in the experiment while the rest of the values can be measured.
I this method, I put the masses in position I and wait until the pendulum reaches equilibrium. I then measure the position and switch the masses to position II. I then measured the period many times and waited until the pendulum reached equilibrium again and measured . With these measurements I calculated . This is within 20% of the accepted value of . This is a good measurement.
Method II: Measurement by Equilibrium Position:
In this method I use the same equation as above. Similarly I measure and and calculate G. First I let the pendulum reach equilibrium with the masses in position I again. As soon as I move the masses to position II I measure the position of the laser on the wall (on a meter stick) every 15 seconds for 45 minutes to an hour. I repeat the process starting with the masses in position II and moving to position I. After plotting the data I could analyze the plots to find and and thus as well as .
Plugging in these values I calculate which is within 20% of the accepted value and within 2% of the value measured in method I. This shows that the greatest error is systematic or in the calibration while my different measurement techniques had smaller error.
Method III: Measurement by Acceleration:
In this method we measure the initial acceleration of the small masses on the pendulum. We have and thus get where . After the pendulum reaches equilibrium in position I, I move the masses to position II and measure the position of the laser every 15 seconds for about 2 minutes. By plotting over I used a linear fit and found the slope. The plot is below.
Using this value I solve for which is not as accurate as my previous measurements. I believe the error here is from the speed of me moving the positions of the masses and measuring the initial acceleration. Any delay can lead to measuring a later (and slightly greater) acceleration which gives us a value of G which is too large.
Overall my measurements gave very close values of the gravitational constant G. If i had to change anything, I would calibrate the equilibrium of the pendulum more accurately. I could only get the laser reflected from the mirror on the wall within 5 cm of the laser reflected from the glass plate. Increasing this accuracy should produce better values of G. This process would take many more hours of guess and check work rotating the zero adjust knob and finding the equilibrium until perfect.